T15.8 Noise Test – To improve the sound quality of single cylinder engines for track workers the sound measuring units may be changed to dBC. This is more consistent with human hearing at the higher volumes called out in the rules. Cheap, commercially available sound meters are generally able to display dBC. The committee is also considering a reduction in the noise level.
Let's take a look at this step by step. First these are the current regulations:
IC3.3 Maximum Sound Level - The maximum permitted sound level is 110 dBA, fast weighting.So currently the maximum permitted sound level for each car is 110dBA (SPL) RMS measured with fast weighting. In order to understand what the impact of this change is, we need to understand what sound level measured in dBA really means. dB in dBA means decibel. We have to dive into the theory about sound pressure levels to see how big the change, especially for single cylinder cars really is. If you want to skip the stuff about sound pressure level theory, just click here.
This is what Wikipedia [http://en.wikipedia.org/wiki/Decibel] has to say about the Decibel:
The decibel (dB) is a logarithmic unit used to express the ratio between two values of a physical quantity (usually measured in units of power or intensity). One of these quantities is often a reference value, and in this case the dB can be used to express the absolute level of the physical quantity. The decibel is also commonly used as a measure of gain orattenuation, the ratio of input and output powers of a system, or of individual factors that contribute to such ratios. The number of decibels is ten times the logarithm to base 10 of the ratio of the two power quantities. A decibel is one tenth of a bel, a seldom-used unit named in honor of Alexander Graham Bell.Wikipedia also offers a further definition:
A decibel (dB) is one tenth of a bel (B), i.e., 1B = 10dB. The bel represents a ratio between two power quantities of 10:1, and a ratio between two field quantities of √10:1. A field quantity is a quantity such as voltage, current, sound pressure, electric field strength, velocity and charge density, the square of which in linear systems is proportional to power. A power quantity is a power or a quantity directly proportional to power, e.g., energy density, acoustic intensity and luminous intensity.The decibel that we are looking for defines sound pressure and thus is considered to be a field quantity. Therefore the sound pressure level is defined as follows [http://en.wikipedia.org/wiki/Sound_pressure]:
So we learned that dB(SPL) RMS [http://en.wikipedia.org/wiki/Root_mean_square] resembles the ratio of a reference sound pressure level (20µPa, Auditory threshold at 1 kHz) and the measured/specified sound pressure level. This is the correct measure for this application as according to several different sources it is the sound level pressure that moves the eardrum and thus it determines the amplitude of its movement. Thus it is also the sound pressure level that determines whether there is a risk of hearing damage. We are not looking for loudness or sound power levels, these are some completely different measures and have no direct connection to the risks of hearing damage or the raw emitted sound pressure level.Sound pressure level (SPL) or sound levelis a logarithmic measure of the effective sound pressure of a sound relative to a reference value. It is measured in decibels (dB) above a standard reference level.
is the reference sound pressure and
is the rms sound pressure being measured.
Sometimes variants are used such as dB (SPL), dBSPL, or dBSPL. These variants are not recognized as units in the SI.[3] The unit dB (SPL) is sometimes abbreviated to just "dB", which can give the erroneous impression that a dB is an absolute unit by itself.The commonly used reference sound pressure in air is= 20 µPa (rms) or 0.0000204 dynes/cm2, which is usually considered the threshold of human hearing (roughly the sound of a mosquito flying 3 m away).
So let us check out the raw numbers: For a moment we will now just forget about the "A" added to dB in the rules: 110dB(SPL) are a ratio of 10^(110/20) => ~316,228. Now you know why they used a logarithmic scale...However, this means that with a reference sound pressure of 20µPa we end up with a maximum sound pressure of 6.324 Pa. This is the allowed maximum, uncorrected sound pressure, 0.5m away from the exhaust in an angle of 45°. This is already enough to damage the hearing, but only, if you put your ears there, which is usually hard/unrealistic unless you are one of the Scrutineers at the Noise Test station.
That means that if you are a track marshal or an official, you will likely be about 5m apart from the exhaust. This would lead to 0.5/5m * 6.324Pa => 0.6324Pa. This still equals 90dB(SPL) RMS. NIOSH, the National Institute for Occupational Safety and Health, states:When measuring the sound created by an object, it is important to measure the distance from the object as well, since the sound pressure decreases with distance from a point source with a 1/r relationship (and not 1/r2, like sound intensity).The distance law for the sound pressure p in 3D is inversely proportional to the distance r of a punctual sound source.
, is measured at a distance
, one can calculate the sound pressure
at another position
,
If a sound reaches 85 dB or stronger, it can cause permanent damage to your hearing. The amount of time you listen to a sound affects how much damage it will cause. The quieter the sound, the longer you can listen to it safely. If the sound is very quiet, it will not cause damage even if you listen to it for a very long time; however, exposure to some common sounds can cause permanent damage. With extended exposure, noises that reach a decibel level of 85 can cause permanent damage to the hair cells in the inner ear, leading to hearing loss.Due to the logarithmic scaling 90dB(SPL) RMS equals a 1.78 times higher pressure than 85dB(SPL). What we can conclude is that the allowed maximum of 110dB(SPL) is already at the borderline of the sound pressure causing hearing damage.
What we have not taken into account so far is the "A" of dBA (SPL). The A indicates a frequency dependant weighting filter which is applied to the measurement. It should describe the sensitivity of the human ear by adjusting/weighting the sound pressure level at different frequencies accordingly. The findings above showed already that hearing protection is not about the perceived loudness, but about the raw sound pressure level. Therefore applying a weighted filter is of no use for this purpose, but it currently is defined in the rules and I will now show how the change from A-weighting to C-weighting affects the hearing protection/allowed sound pressure levels for single cylinder and 4 cylinder cars.
There are different frequency-dependant weighting curves for sound pressure levels. All of them should somehow resemble the sensitivity of the human hearing. That also means they are pretty useless for hearing protection means, because the physical damage due to pressure levels knows no sensitivity correction. The fact that the A-weighting is so often used is due to a cartel formed by industry in order to use the most forgiving weighting for noise emission and protection and this in fact is the A-weighting. This diagram [http://commons.wikimedia.org/wiki/File:Weighting_curves.png] shows the different weighting methods:

As you can see, the A-weighting has the biggest damping, especially for lower frequencies. As this is also given in dB, we can just add/subtract the values (you remember calc rules for logarithmics, right?).
In order to figure out which attenuation is correct, we need the major frequency in the spectrum of the measured signal.
For IC engines the major frequency is the ignition frequency as the sound pressure is released by the exhaust valves opening in this frequency. A single cylinder engine is usually measured at about 6,500RPM (Yamaha YZF450) for example. 6,500RPM equal an ignition frequency of about 54Hz (1 cylinder * 6,500 / 2 / 60).
A four cylinder engine is measured at 10,500RPM (Suzuki GSX-R 600), which equals an ignition frequency of 350Hz (4 cylinders * 10,500 / 2 / 60).
Thus the resulting attenuation for a single cylinder engine a its major frequency is ~ -32dB and ~ -4dB for four cylinder engines taken from the A-curve of the diagram above. So when looking at uncorrected measurements, single cylinders just meeting the 110dBA(SPL) are emitting about 142dB(SPL) and four cylinder engines are emitting 114dB(SPL). This easily shows why the singles seem so much louder...because they actually are. It is however an exaggeration of the situation as the emitted frequency spectrum is broader and not a sharp needle in the spectrum. Thus the difference will more likely be about 1 to 2dB(SPL) for four cylinders and 8 to 9 dB(SPL) for single cylinder cars. These numbers come from measurements which I have personally taken during noise tests with dBA and dBC weighting.
Switching to the C-weighting curve turns the damping more or less off. Single cylinders will be damped by about -2dB, while four cylinders will not be damped at all. That means that this will create a big task for the single and two cylinder teams, while the four cylinder teams probably only have to make minor adjustments, if any. I would favour changing to a totally uncorrected measurement, but there are not many devices available that give uncorrected readings. Most devices allow to measure in dBC though, so this is a good compromise.
This change also means that the track marshals and officials will only be "punished" with 112dB(SPL) which leads to 0.8Pa in 5m distance compared to a maximum of about 118dB(SPL) now equalling 1.59Pa in the same distance. So it will be half as loud. As the RMS value is taken, it will also be fairer with respect to the emitted power in general, as this levels the emitted sound pressure level over frequency and time.
And before you ask:
It was me who proposed to change from dBA to dBC, so blame me, if you want to.
You may use a smartphone app to calculate an FFT [http://en.wikipedia.org/wiki/Fast_Fourier_transform] to find out the spectrum of your engine and determine how much you will be affected by this rules change. However, never trust the dB(SPL) values given by these apps as the accuracy is very limited by the smartphone's hardware.
Please note:
If you think that any content in this post is worth discussing, please do so in the FSAE.com forum and not in the comment section of this blog, to make sure that everyone can benefit from the contents of the discussion, even if he/she is not reading this blog.

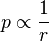


No comments:
Post a Comment